The Trigonometric Laws of Sines and Cosines
Created | Updated Mar 21, 2003
It is not the intention of this Researcher to include the sum total of all known trigonometry in one guide entry. There are, however, a few fundamental concepts in this area of study that are worthy of note simply for the fact that they are mathematically elegant, and that their practical uses in a myriad of different fields goes without saying.
It would not seem worthy to simply state these concepts without some attempt to argue their validity, so an elementary knowledge of the basics of right-angle trigonometry is required in order to appreciate the meaning of the following justifications. The formulae generated by these concepts, however, require little more than a pocket calculator.
In utilising the Laws of Sines and Cosines, it is usually assumed that problems are presented wherein at least three of the six quantities (the six being the three side lengths together with the three angles) for the various triangle measures are given, or at least implicitly obtainable from the given information. This hardly detracts from their real-world uses, in which triangles exist across rivers, through outer space, or wherever people choose to connect the dots that they can't necessarily reach from a physical standpoint. In these cases, surveyors and/or scientists are able to measure some, but not all, of the quantities they need. They are usually able to measure just enough to let trigonometry do its thing... and with that, what follows are a few examples of the things it can do.
The Law of Sines can be used to determine completely the sizes of the sides and the angles in a given triangle, provided the given triangle information is:
The sizes of any two angles and the length of any one side
or
The size of any one angle and the length of the side opposite it, together with one other side-length
However, in scenario (2) exists the possibility of no triangles, one triangle, or even two triangles satisfying the given information. This is the aptly-named 'ambiguous case' in trigonometry.
Scenarios in which the Law of Sines is not a powerful enough tool for completely determinining a triangle's measurements occur if the given information about the triangle is:
The sizes of the three angles
The lengths of the three sides
or
The lengths of any two sides and the angle they form
In scenario (1) there are an infinite number of solutions based upon the geometric concept of similar triangles, which occur when corresponding angles of differently-sized triangles are congruent1. For cases (2) and (3) the Law of Cosines must first be utilised in order to determine enough additional information about the triangle in order to complete the problem using the easier Law of Sines.
Notation
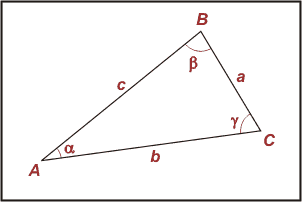
The process of labelling the vertices, sides, and angles of triangles with various letters for notational and referral purposes in trigonometry is kept consistent from triangle to triangle. Since the Laws of Sines and Cosines are written with this notation in mind, it is best to introduce the various letters before the Laws.
Let triangle ABC be any triangle. In more mathematically explicit language, for the purposes intended here: A, B and C are three given points in a two-dimensional space, not all colinear2, such that each point is at a vertex of the triangle formed by pairwise connection of the given points with line segments.
Let the lower-case Greek letters α, β and γ refer to the angles within triangle ABC with vertices at the corresponding upper case English letters.
Let lower-case letters a, b and c refer to the lengths of the sides of triangle ABC, where side a is opposite angle α, side b is opposite angle β, and side c is opposite angle γ (see Diagram One).
Assumptions
In proving these laws, we will make a number of assumptions:
The triangle does not contain a right-angle. If it does, we may use the trigonometrical formulae for right-angled triangles, rather than the slightly more complex Sine and Cosine Laws.
The triangle in question is in R² - that is, a 2-dimensional Cartesian Plane3 (sometimes known as an '(x,y) plane'). The Sine and Cosine Laws are only valid in this setting - they will not work if the triangle is drawn on the surface of a sphere, for example.
The Law of Sines
One of the two fundamental relationships which holds true for any given triangle is the Law of Sines. This law asserts that:
a/(sin α) = b/(sin β) = c/(sin γ)
Or equivalently:
(sin α)/a = (sin β)/b = (sin γ)/c
Proof
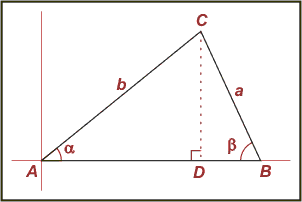
Let triangle ABC be oriented in such a way that vertex A coincides with the origin and vertex B lies on the positive x-axis. In this position, α is now an angle in 'standard position' (a trigonometric term for an angle with one ray of the angle coinciding with the positive x-axis in an (x,y) plane), with vertex C either lying above or below the x-axis (see Diagram Two).
Without loss of generality, it may be assumed that C lies above the x-axis (for if it did not, the reflection C' of point C across the x-axis could be utilised, and the validity of the proof for triangle ABC' would extend to the 'mirror-image' congruent triangle ABC). Also note that segment AC is equivalent to side b in triangle ABC, and segment BC is equivalent to side a.
Letting D be the point on the x-axis that is also on the same vertical line as vertex C, both triangles ACD and BCD are right triangles (triangles containing 90° angles) with right angles each at vertex D.
Using concepts in the trigonometry of right triangles, in triangle BCD we find that sin β = CD/BC = CD/a, hence CD = a*sin β.
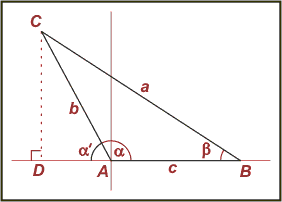
At this stage, we are in one of two possible situations. If α<90°, α will be interior to (ie inside) the triangle ACD. In this case, we get sin α = CD/AC = CD/b, hence CD = b*sin α. However, if α>90°, the interior angle of ACD at vertex A will be an angle α', satisfying α + α' = 180° (see Diagram Three). Then, using the subtraction formula for sine, sin α' = sin (180° - α) = [(sin 180°) * (cos α)] - [(cos 180°) * (sin α)]. But sin 180° = 0 and cos 180° = -1, thus sin α' = sin α, and the result CD = b*sin α is again obtained.
From these results, we see that a*sin β = CD = b*sin α. Dividing both sides by a*b gives us (sin α)/a = (sin β)/b - the desired result, which can then be generalized to the three-part Law of Sines based upon the ability to re-orient the given triangle into the necessary position. QED4.
The Law of Cosines
The Law of Cosines is the second of two fundamental relationships which holds for all arbitrary triangles. It is more complex than the Law of Sines, however one saving grace in its favour is that once the Law of Cosines is utilised once to determine one additional piece of information about a triangle, then enough information will be known about the triangle at that point to be able to use the more simplistic Law of Sines to determine the remainder of the triangle.
Using the same notation as before, the Law of Cosines asserts that:
a² = b² + c² - 2bc*cos α.
This may also be stated in the following forms:
b² = a² + c² - 2ac*cos β, and c² = a² + b² - 2ab*cos γ.
Three forms are given, even though one would suffice. This is done to bring forward the fact that the notation itself is irrelevant, it is the relationship of the four variables in the equation that remains consistent. In regular language, the Law of Cosines states that:
The square of any one side equals the sum of the squares of the other two, less two times their product times the cosine of the angle opposite the first side.
Reviewing the pattern of each of the 3 versions of the Law will make this clear, and it is quite often easier to commit this lengthier Law to memory by memorising the relationship as opposed to the three distinct formulae.
Proof

The first version of the Law of Cosines will be proven, and without loss of generality, that result may then be extended to the other two variations. Let triangle ABC be situated in an (x,y) plane in the same manner that was established in the first two paragraphs of the proof for the Law of Sines. Again, let D be the point on the x-axis and on the same vertical line as C. Let the co-ordinates of C be represented by the co-ordinate pair (d,e) (see Diagram Four).
Using concepts in the trigonometry of right triangles, in triangle ACD (using the chosen variables for the co-ordinates of point C) we find that:
cos α = d/b, and sin α = e/b,
from which we find the following values for co-ordinates d and e:
(d,e) = (b*cos α,b*sin α).
Since segment AB on the positive x-axis corresponds to side c and A lies at the origin, then B can be said to have co-ordinates (c,0). Segment BC corresponds to side a, and can be determined by using the Pythagorean distance formula5 on points B and C:
| a² | = | d(B,C)² = (d - c)² + (e - 0)² | (Using the distance formula) | |
| = | (b*cos α - c)² + (b*sin α)² | (Substituting in the values found for d and e) | ||
| = | b²cos²α - 2bc*cos α + c² + b²sin²α | (Expanding the brackets) | ||
| = | b²(cos²α + sin²α) + c² - 2bc*cos α | (Re-grouping) | ||
| = | b² + c² - 2bc*cos α | (From the trigonometrical identity cos²x + sin²x = 1) | ||
| QED. | ||||
It is sometimes more useful to have the Law of Cosines in a form in which the solution for one of the angles is in terms of the three sides. As an example of how the Law of Cosines can be manipulated, if one solves for the angle α in the first given version of the Law of Cosines, the formula becomes:
α = arccos[(b² + c² - a²)/2bc]
where 'arccos' refers to the inverse cosine function (alternatively notated as cos-1). In this form, the values of the three sides are easily plugged and the value of α is quickly determined with the use of a standard calculator.
However...
There is a caveat in using the Law of Cosines. Namely, if the given pieces of information for a triangle are lengths of the three sides, it is imperative that the first angle found be the angle opposite the longest side.
The reason for this is that there is always the possibility that one of the angles in the triangle will be obtuse (ie more than 90°). If there is going to be any obtuse angle in any triangle (at least in Euclidean geometry), then it will be opposite the longest side. If one of the smaller angles is found first and the Law of Sines is used to finish the problem, there exists the possibility of obtaining incorrect solutions if one of the angles of the triangle turns out to be obtuse.
Diagrams provided by Archangel Dr Justin.

